Hexaflexagons, Hexahexaflexagons, Dodecahexaflexagons and Beyond!

Preambulist [CC0], from Wikimedia Commons

Flexagons came to public attention when the late great Martin Gardner (who wrote the excellent Mathematical Games column in Scientific American) did a column on them in 1956 which included a brief history:

Martin Gardner's Flexagon Article

 
One of the clearest descriptions of the procedure for making a hexaflexagon:

wikiHow:  HOW TO MAKE A FLEXAGON 

I made a hexaflexagon of this sort from stiff card stock paper.  At first I thought that this paper might make flexing difficult, but found that it actually makes it easier to flex.  Here is a brief video in which I flex through the three sides: yellow, purple and green:

A great flexagon resource page has been provided

 by Jürgen Köller:

Jürgen Köller's Flexagon Page 

Making a Hexahexaflexagon

A hexahexaflexagon is a hexagonal flexagon with six edges which can reveal six faces.  It can be made from a single strip of paper with 19 equilateral triangles.  For mine I used the following template printed twice on 8.5x11 card stock.  I then glued the two segments together by overlapping one triangle to give the needed 19 (which includes one as a flap for gluing).  This template gives a strip that is the width of a standard roll of clear packing tape, so before folding you can cover both sides with packing tape for extra strength:

The following video presents a good numbering system for making a hexahexaflexagon:

 The next video makes clear the final crucial phase of constructing a hexahexaflexagon:  the orientation and gluing of the flaps:

St Mary's University provides a PDF template for making this particular hexahexaflexagon:

St Mary's University also has a great Web Page on Flexagons.

Here is a brief flexing tour of my hexahexaflexagon that I covered with packing tape before folding:

 

For the above hexahexaflexagon you can make the following Feynman diagram of all the possibilities paired according to the colors of the front and back of the flexagon at that point.  You will notice that each pairing of front and back is unique, so taking up your hexahexaflexagon you will immediately know where you are on the diagram and how to flex to get anywhere you wish to go. The circles in the bottom right simply show all six colors available:

In the process of making a hexahexaflexagon, Vi Hart, the queen of flexagons describes the history of flexagons including Feynman diagrams that map the flexing relations and the Tuckerman traverse that provides a topological path to all six sides of the hexahexaflexagon:

Making a Dodecahexaflexagon

The dodecahexaflexagon is a particularly fascinating elaboration of the basic hexaflexagon.  It has twelve different faces.  Michael Anttila provides an excellent walk through of the twelve faces and twenty one states of the dodecaflexagon:

 Michael Anttila also provides a step by step video on the making of his marvellous dodecahexaflexagon:

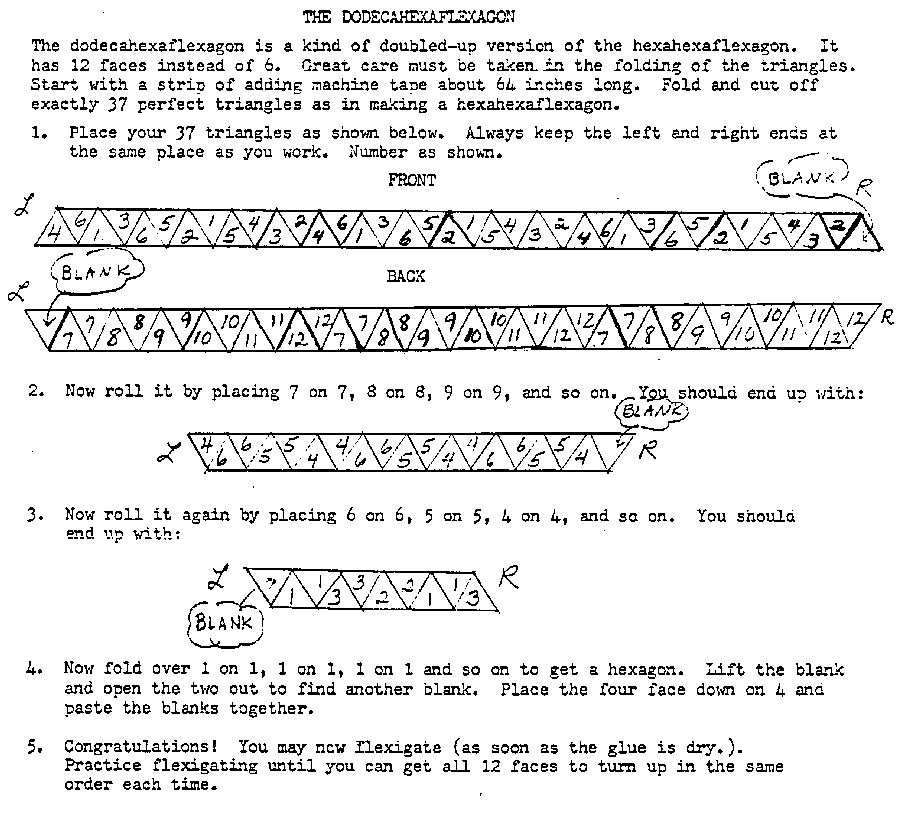
For my dodecahexaflexagon I followed the following excellent instructions from David Pleacher:

I used card stock for the base and the same template as I used for my hexahexaflexagon above.  Instead of numbers I used smaller colored paper triangles in twelve colors and covered the whole strip with clear packing tape before folding.  Here is the result:

 
 I also made a Feynman diagram for my dodecahexaflexagon.  Each circle has the colors of the front and back of the flexagon at that point.  I named the outside areas after the related colors which are the hardest ones to flex to.  All twelve colors used are show in the bottom right:

 And beyond the dodecahexaflexagon, there are many other flexagon variations to explore, such as the amazing 3D origami moving flexagon, presented by Joe on the YouTube Channel:  How to Origami... 

I followed Joe's excellent instructions and made my first 3D Hexaflexagon.  I decided to make a number of models and for this reason made a template that made accurate folding easier:

In his instructions, Joe just tucked one side into the other, but I decided to make this easier and the result less thick and more flexible by creating a glue flap instead.  I printed this template on card stock, cut it out and made my 3D Hexaflexagon with four sides:  Red, Blue, Yellow and Black:

I made another 3D Hexaflexagon using brightly colored paper glued to cardstock.  I then covered the whole sheet of paper with clear packing tape.  The packing tape made folding more difficult and it took longer to flex it in, but the result was quite good:

Icosikaitetrahexaflexagon!

The icosikaitetrahexaflexagon has 24 sides, or 24 different faces to flex to.  Here is a brief description from teacher Danny Doucette:

 

 Richard Dibbs and his friends present an excellent video of how to make the icosikaitetrahexaflexagon:

The numbering for the triangles is given in the video description:

 

Finally, cbx33 presents a fascinating and complete walk through of the icosikaitetrahexaflexagon with progress shown on an inset Feynman diagram: